Knot theory, low dimensional topology

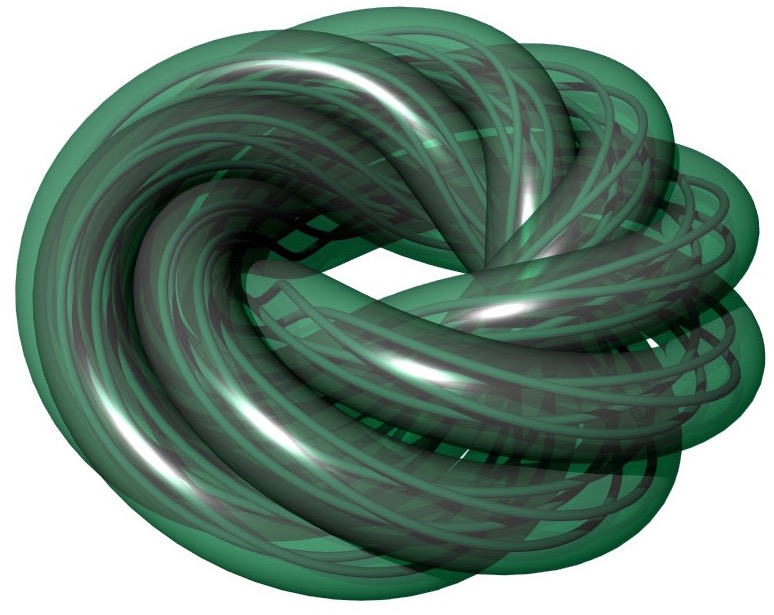
I study how objects interact in space. A knot is a curve that lives in 3 dimensions that cannot pass through itself. As a knot theorist, I want to understand what makes knots different from each other. I also look into how they can be arranged, with respect to a surface, in a minimal way. Specifically, I am interested in bridge spectrum and an invariant called the straight number of a knot, which is an invariant I defined.

Learn more

Mathematics education

Teaching is a passion of mine. I have been lucky enough to have had the opportunity to teach a variety of classes: trigonometry, lecturing calculus I, and a class for future teachers, just to name a few. I have created and organized a 3D modeling and printing seminar. The goal of the seminar is to introduce undergraduates, graduates, and faculty to the basics of 3D modeling and printing, then move onto some more advanced concepts.

Learn more

Geometry and Topology of 3-manifolds workshop

Service to the unit and the university has always been a focus of mine. At OIST, I was a local organizer of an International workshop in my research area. At Gettysburg, I did outreach to high school students to get them interested in math. While at UNL, I was elected to serve on the graduate student advisory board, an organization meant to be a liaison between the graduate students and the department. I was also a local organizer for the MoSAIC Festival, which is sponsored by the Mathematical Sciences Research Institute, the Bridges Organization, and the Simons Foundation.

Learn more

Projects and information

Below are a few projects I have been working on, many with collaborators and all my job application materials, including my CV and publication list. Some links do not work yet, as we are still under construction.

Geometry and Topology of 3-manifolds workshop

We are organizing and hosting an international workshop here at OIST, May 25-18, 2018. Looking forward to seeing you all there!

Learn more

Straight knots, random straight links, and snail links

Some of my current research topics are to investigate the different properties of straight number, a knot invariant I defined. There is an interesting relation between this invariant and the hyperbolic volume.

Learn more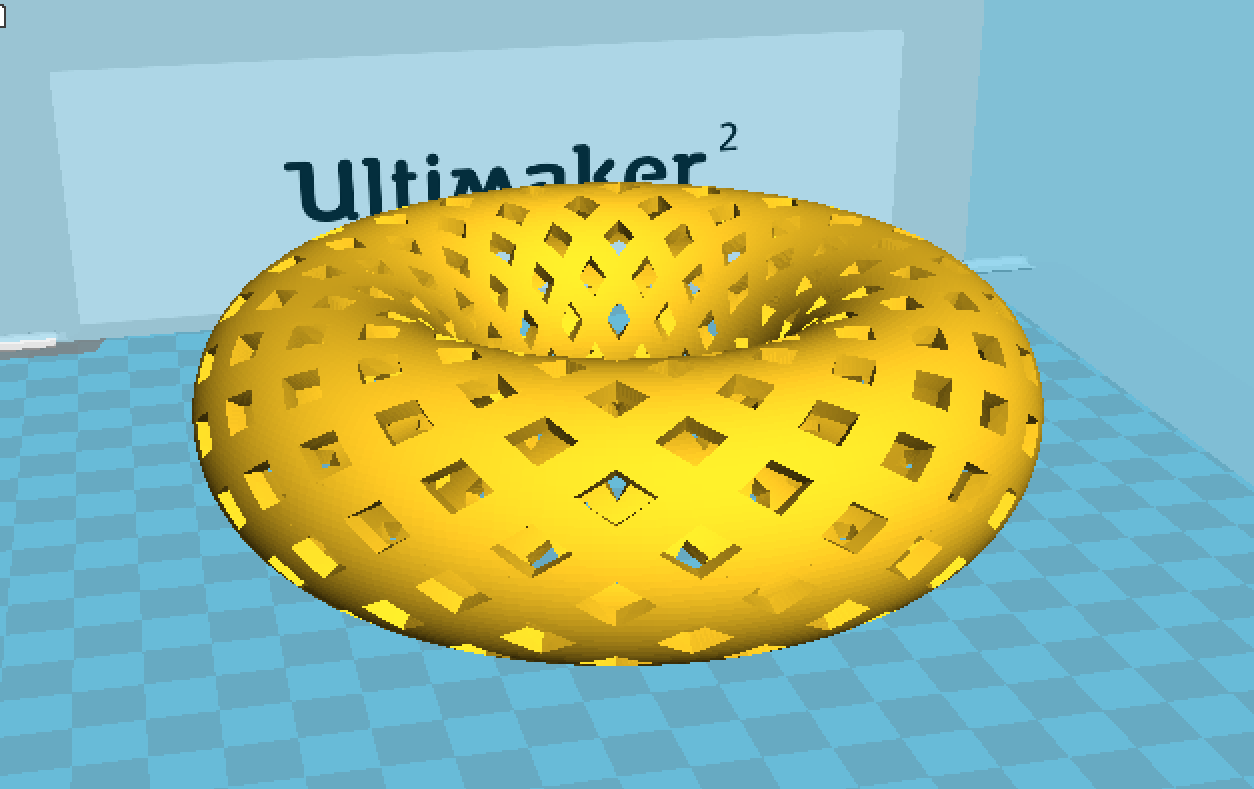

Downloadable

The things I have made that you might want to download. From research papers, to talks I have given, to files to 3D print, you will find it here.

Learn more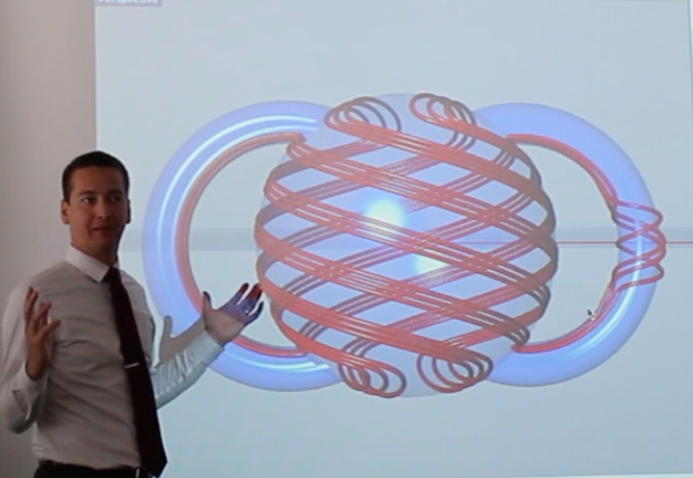

Job Materials

Here is where I keep my CV, teaching statement, research statement, and publication list.

Learn more